VVT13 - Hollow cylinder with fixed temperature and convective boundary conditions
| Solution | Test case |
|---|---|
| Finite volume method | SVTEST17 |
| Finite element method | SVTEST243 |
Description
The purpose of this test case is to determine the temperature distribution through the thickness of a hollow cylinder. The heat is transferred by conduction through the thickness of the cylinder wall and by convection inside the cylinder.
Geometry
The hollow cylinder is defined with an inner diameter of 600 mm and an outer diameter of 700 mm. The axisymmetric modeling technique is used, which requires the sketch on the XZ plane. Cylinder thickness is 50 mm, therefore a plate is sketched with the length of 50 mm and height of 10 mm, starting from the inner diameter of the cylinder. A bounded plane is attached to this plate.
Simulation model
This model uses the Axisymmetric Thermal solution type.
A 2D mesh of quadrilateral thin shell elements is generated on the surface of the plate, using 10 mm element size.
The meshed elements have the following material and physical properties:
- Thermal conductivity: k = 1.0 W/m·°C
- Mass density: ρ = 1kg/m3
- Thickness: not required for the axisymmetric thermal analysis
The following boundary conditions are applied:
- Temperature constraint on the outside of the cylinder with a value of To = 20 °C.
- Convection to Environment constraint on the inside edge of the cylinder with a heat transfer coefficient hi = 30W/m2·°C and an environment temperature Ti = 100 ºC.
The following figure shows the mesh and the location of the convective and fixed temperature boundary conditions.
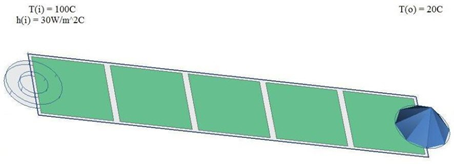
The following solution options are set:
- Solution Type=Steady State
- Results Options: Select the heat flux and temperature at nodes and elements
The default solver parameters are selected.
Theory
The heat transfer rates by conduction through the cylinder thickness and by convection along the inside surface of the hollow cylinder must be determined [4], [5], [6] using the following equations:
From these results, the inner surface temperature is determined by:
Using the inner surface temperature, the through-wall temperature distribution for the hollow cylinder can be determined using the following equations;
Results
The table below compares the node temperatures computed by the thermal solver with theoretical values.
| Finite volume method | Finite element method | ||||
|---|---|---|---|---|---|
| Rcyl(mm) | Ttheory (ºC) | Tsim (ºC) | Error (%) | Tsim (ºC) | Error (%) |
| 300 | 66.49 | 66.49 | 0 | 66.49 | 0 |
| 320 | 47.03 | 47.10 | 0.15 | 47.03 | 0.01 |
| 340 | 28.74 | 28.81 | 0.24 | 28.74 | 0.01 |
| 350 | 20 | 20 | 0 | 20.00 | 0 |
The following table shows the heat transfer rates by conduction through the cylinder thickness.
| Finite volume method | Finite element method | |||
|---|---|---|---|---|
| Rcyl (mm) | qr, (W/m) | Error (%) | qr, (W/m) | Error (%) |
| 305 | 1895.44 | 0.009 | 1895.02 | 0.014 |
| 315 | 1895.42 | 0.008 | 1895.03 | 0.013 |
| 325 | 1895.40 | 0.006 | 1895.03 | 0.013 |
| 335 | 1895.37 | 0.005 | 1895.03 | 0.013 |
| 345 | 1895.33 | 0.003 | 1895.02 | 0.014 |
