VVT10 - Transient heat flow in a semi-infinite solid
| Solution | Test case |
|---|---|
| Finite volume method | SVTEST13 |
| Finite element method | SVTEST239 |
Description
The purpose of this test case is to determine how long it takes for a semi-infinite solid at a temperature of 200 °C to reach the temperature of 120 °C at a depth of 4 cm if the temperature at the surface of the solid is suddenly dropped to 70 °C. A single elements row can be used to model the semi-infinite solid, since this is a one-dimensional problem.
Geometry
A solid geometry part is created by extruding a 5 mm by 5 mm square cross-section to a length of L = 300 mm.
Simulation model
The regular mesh is made of sixty 5 × 5 × 5 mm3 hexahedral solid elements along the length of the extrusion. The ends of the extrusion are selected as the source and target faces. An additional quadrilateral element with zero thickness is manually assigned to the end of the solid mesh. This element is created in order to calculate the total heat passing through the surface of the semi-infinite solid.
The meshed elements have the following material and physical properties:
- Mass density: ρ = 2700 kg/m3
- Thermal conductivity: k = 215 W/m·°C
- Specific heat at constant pressure: Cp = 948 J/kg·°C
The following boundary conditions are applied:
- Initial Conditions constraint: Initial Temperature on the solid geometry with a value of Ti = 200 °C.
- Temperature constraint on the end of the solid with a value of To = 70 °C.
- Report: Cumulative Absorbed Heat on the single quadrilateral element.

The following solution options are set:
- Solution Type=Transient (the model is simulated for 38 seconds, constant time steps of 1 ms, time interval t = [37.5,38.0] seconds, results are sampled every 10 ms).
The default solver parameters are selected.
Theory
The partial differential equation for the temperature distribution, T(x,t) in the semi-infinite solid is defined as:
where = 8.4 × 10−5 m2/s.
The boundary and initial conditions are found as:
and the solution is:
Solving for t given x = 0.04 m:
The flux at the surface is obtained by differentiating
and invoking Fourier’s law:
Integrating up to t = 37.72 seconds:
Results
A plot of nodal temperature across the time steps is generated in order to obtain an estimate of when the temperature at a depth of 4 cm reaches 120 °C. As shown in the following figure, this occurs at t = 37.67 seconds.
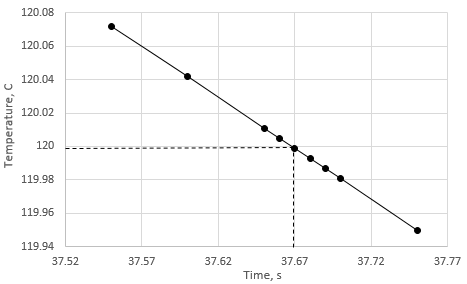
Referring to the [Solution_name]_report.log file generated by the thermal solver, the heat, absorbed by the quadrilateral element on the interval t = [0 37.67] seconds, is 5.211 × 108 mN·mm or 521.1 J. These values are summarized in the table below.
The time predicted by the thermal solver is in agreement with theoretical value. There is less agreement between computed heat with theoretical value. A finer mesh near the surface of the semi-infinite solid could remedy this.
| ttheory(s) | tsim (s) | Error (%) | Qo,theory (J) | Qo,sim (J) | Error (%) |
|---|---|---|---|---|---|
| 37.72 | 37.67 | 0.13 | 528.25 | 521.1 | 1.4 |
