VVC9 - Turbulent natural convection on a vertical plate
| Medium | Geometry | Temperature constraint | Test case | |
|---|---|---|---|---|
| Air | 1 | Case1 | SVTEST107 | |
| Case2 | SVTEST108 | |||
| Case3 | SVTEST109 | |||
| 2 | Case4 | SVTEST110 | ||
| 3 | Case5 | SVTEST111 | ||
| Water | 1 | Case1 | SVTEST112 | |
| Case2 | SVTEST113 | |||
| Case3 | SVTEST114 | |||
| 2 | Case4 | SVTEST115 | ||
| 3 | Case5 | SVTEST116 | ||
Description
This case examines the turbulent natural convection on a vertical plate for two mediums: water and air. Average Nusselt numbers are compared to the theoretical results of Churchill and Chu [44].
Geometry
The geometry includes a vertical plate with a length = L, width (W) = L/6, height (H) = L*3 and depth (D) = 500 mm. All dimensions other than the thickness (depth) are proportional to the plate length, which allows easily to set up different combinations of plate lengths and boundary conditions.
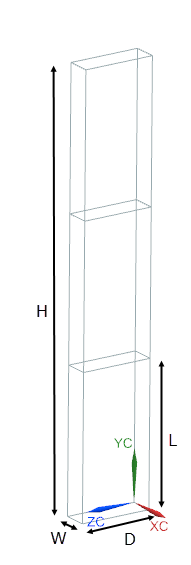
The following table lists the various dimensions used in the model.
| Geometry | L,m | H,m | W,m |
|---|---|---|---|
| 1 | 1 | 3 | 0.1667 |
| 2 | 1.45 | 4.35 | 0.2417 |
| 3 | 2.60 | 7.80 | 0.4333 |
Simulation model
The same mesh density per edge is used for both: water and air models. A mapped mesh is used so that the number of elements and nodes remain constant regardless of the value given for the plate. The following table shows the number of elements per each edge. The plate surfaces on the X-Y plane at Z=0 are meshed with material type generic isotropic steel and considered as thin shell with 10 mm thickness, while all other bounding surfaces are meshed with the fluid material type.
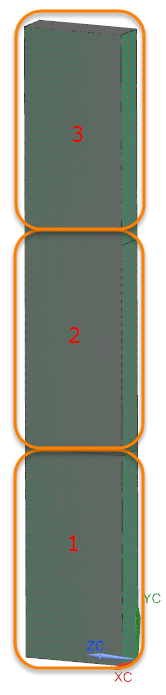
The following table describes the mesh controls used in the model.
| Section | Position | Number of elements | Bias factor | Edge fraction |
|---|---|---|---|---|
| 1 | X | 2 | - | - |
| Y | 40 | 1.031 | 1 | |
| Z | 60 | 1.0087 | 1 | |
| 2 | X | 2 | - | - |
| Y | 80 | - | - | |
| Z | 60 | 1.0087 | 1 | |
| 3 | X | 2 | - | - |
| Y | 40 | 1.031 | 1 | |
| Z | 60 | 1.0087 | 1 |
The fluid is modeled using air and water with the following properties:
- Mass density: air - ρ = 1.2041 kg/m3, water - ρ = 1000 kg/m3
- Thermal conductivity: air- k = 0.0263 W/m·K, water - k = 0.603 W/(m·K)
- Dynamic viscosity: air - µ = 1.85e-5 kg/m·s, water - µ = 0.001006 kg/(m·s)
- Specific heat at constant pressure: air - Cp = 1007 J/(kg·K), water - Cp = 4187 J/(kg·K)
- Coefficient of thermal expansion: air - β = 0.00341 1/°C, water - β = 0.000182 1/°C
- Gas constant: air - R = 287 J/(kg·K), water - NA
The following boundary conditions are applied:
- Flow Surface: Boundary Flow Surface with Obstructions on the surface of all the three X-Y faces at Z=0 mm, using the Wall Function option with smooth wall friction and convection properties set to Automatic
- Flow Surface: Boundary Flow Surface on the surface of all the three X-Y faces at Z=500 mm, using the Slip Wall option with convection properties set to Automatic
- Flow Boundary Condition: Opening on the top and bottom surfaces (two X-Z faces) with ambient external conditions
- Symmetry Plane on the surfaces lying in the Y-Z plane
- Temperature constraint on the surface of all the three X-Y faces at Z=0 mm based on the values in the following table.
| Case | Geometry | Model 1 - Air Tw, °C | Model 2 - Water Tw, °C |
|---|---|---|---|
| 1 | 1 | 21.5 | 20.4 |
| 2 | 1 | 28 | 22 |
| 3 | 1 | 65 | 24 |
| 4 | 2 | 100 | 26 |
| 5 | 3 | 200 | 28 |
This model uses the Advanced Thermal-Flow solution type with the following solution options:
- Turbulence Model: Standard K-Epsilon
- Buoyancy: selected
- Solution Type: Steady State
- 3D Flow: wall function, smooth with friction
- Ambient Conditions: fluid temperature = 20 °C, pressure 0.101351 MPa, gravity = 9810 mm/s2 in -Y direction
The following non-default solver parameters are used:
- 3D Flow Solver: Physical steady-state with time step = 2 s
- 3D Flow Solver: Maximum Residuals = 1e-4
- 3D Flow Solver: Buoyancy Model=Boussinesq
- 3D Flow Solver: Advection Schemes group: momentum energy, turbulence model = first-order
- Coupled Solver: max temperature change = 0.001 Δ°C, global imbalance fraction = 0.0001
Theory
The theory of Churchill and Chu [44] is used as a basis of comparison for the solver results. The Nusselt number is computed using the following formulas:
The Rayleigh number is computed using the following equations:
where:
- Tamb is the ambient temperature.
- Tw is the plate temperature.
- L is the plate length.
- α is the thermal diffusivity.
The Prandtl number is calculating using the following expression:
where:
- μ is the fluid viscosity.
- ρ is the fluid density.
Results
The following tables compares five different combinations of plate length and temperature for air and water models used to examine the desired range of Rayleigh numbers.
Model 1 - Air
| Case | Geometry | L, m | RaL | Pr | Nucorr | Qw | Ap | h | Nusim | Nu difference, % |
|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 1 | 3 | 4.065E+09 | 0.708 | 189.97 | 1.01E+00 | 0.50 | 1.34 | 152.82 | 19.56 |
| 2 | 1 | 3 | 2.168E+10 | 0.708 | 322.31 | 1.02E+01 | 0.50 | 2.55 | 290.53 | 9.86 |
| 3 | 1 | 3 | 1.219E+11 | 0.708 | 560.10 | 1.11E+02 | 0.50 | 4.9 | 561.61 | 0.27 |
| 4 | 2 | 4.35 | 6.609E+11 | 0.708 | 967.08 | 4.88E+02 | 1.05 | 5.79 | 959.02 | 0.83 |
| 5 | 3 | 7.8 | 8.573E+12 | 0.708 | 2230.57 | 4.38E+03 | 3.38 | 7.2 | 2135.3 | 4.3 |
Model 2 - Water
| Case | Geometry | L, m | RaL | Pr | Nucorr | Qw | Ap | h | Nusim | Nu difference, % |
|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 1 | 3 | 1.331E+11 | 6.99 | 721.72 | 30.20 | 0.50 | 150.97 | 751.09 | 4.07 |
| 2 | 1 | 3 | 6.655E+11 | 6.99 | 1216.36 | 238.2 | 0.50 | 238.15 | 1184.84 | 2.59 |
| 3 | 1 | 3 | 1.331E+12 | 6.99 | 1524.61 | 578.2 | 0.50 | 289.04 | 1438.02 | 5.68 |
| 4 | 2 | 4.35 | 6.086E+12 | 6.99 | 2506.73 | 2088 | 1.05 | 330.99 | 2387.73 | 4.75 |
| 5 | 3 | 7.8 | 4.678E+13 | 6.99 | 4900.32 | 10330 | 3.38 | 382.06 | 4942.02 | 0.85 |
