Across gap convection correlations
Across gap convection correlations calculates heat transfer coefficients for the free convection between closely spaced parallel walls based on their orientation, geometry, and walls temperatures.
Free convection between horizontal parallel plates
The parallel plate channel correlation models natural convection where an open channel is horizontal, vertical, or inclined to gravity.
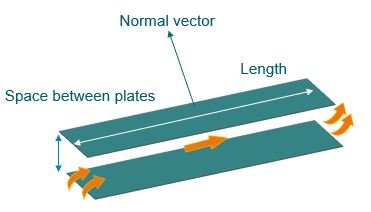
When the hot plate is on top and the cold plate is on the bottom, the following correlations are used [4]:
where:
- ẟ(X) = X for X > 0
- ẟ(X) = 0 for X < 0
Free convection between vertical parallel plates
| Pr<1 | Pr>1 |
The Nusselt number for the vertical parallel plate is computed based on the Raleigh number and laminar, turbulent and transition boundary layer regimes as follows [4]:
where:
- L is the distance between the plates.
- H is the height of the channel.
Free convection between inclined parallel plates
When the cavity is inclined, the Nusselt numbers are obtained for different conditions depending on the angle and parameter HL=H/L, where H is the length of the plates and L is the distance between the plates.
| τcrit=25 | HL < 1 |
| τcrit=53 | 1 < HL ⩽ 3 |
| τcrit=60 | 3 < HL ⩽ 6 |
| τcrit=63 | 6 < HL ⩽12 |
| τcrit=70 | 12 ⩽ HL |
When the bottom plate is hotter than the top plate, then τ = 90°-θ.
When the bottom plate is cooler than the top plate, then τ = 90°+θ.
The following parameters are used [4]:
where:
Nuct, NuL, NuT is the Nusselt numbers computed using the free convection between vertical parallel plate correlation.
The following Nusselt numbers are used depending on the angle and plate parameters.
| Nusselt number | Parameters |
| HL > 12 and τ < τcrit | |
| HL < 12 and τ < τcrit | |
| τ > 90° and τ > τcrit | |
| 90° < τ < 180° and τ > τcrit |
Free convection between inclined parallel plates and fluid ducts for convective thermal coupling
The following correlation is used to model natural convection from walls of an open inclined channel and fluid ducts for convective thermal coupling. If the inclined channel is horizontal, then the horizontal cavity correlation is used.
The Rayleigh number for inclined cavity is [4]:
where
- L is the distance between the walls.
- H is the length of the channel.
- θ is the angular deviation from the vertical plane in degrees.
The Nusselt number used to compute the heat transfer coefficient is:
Between concentric spheres

The thermal solver computes the heat transfer coefficient of the flow convecting from the outer sphere to the inner sphere, assuming the convection happens on the surface of the outer sphere.
The Rayleigh number of concentric spheres is [4]:
where D0 is the outer sphere diameter and Di is the inner sphere diameter.
The function used to compute the heat transfer coefficient is:
The heat transfer coefficient is computed as:
where k is the thermal conductivity of the fluid.
Between long concentric horizontal cylinders
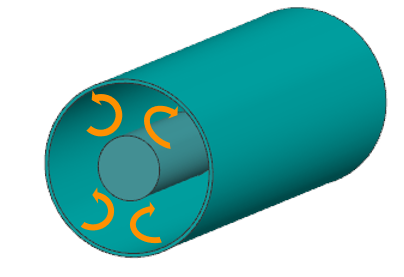
The thermal solver computes the heat transfer coefficient of the flow convecting from the outer cylinder to the inner cylinder assuming the convection happens on the surface of the outer cylinder.
The Rayleigh number for concentric horizontal cylinders is [4]:
where D0 is the outer sphere diameter and Di is the inner sphere diameter.
The function used to compute the heat transfer coefficient is:
The heat transfer coefficient is computed as:
